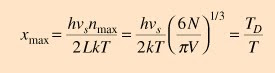Broken Translational Invariance in the Solid State. Simple Energetics of Solids. Phonons: Linear Chain. Quantum Mechanics of a Linear Chain. Statistical Mechnics of a Linear Chain. The 1D chain Discrete Translational Invariance: the Reciprocal Lattice, Brillouin Zones, Crystal Momentum. Phonons: Continuum Elastic Theory. Debye theory. More Realistic Phonon Spectra: Optical Phonons, van Hove Singularities. Lattice Structures. Bravais Lattices. Reciprocal Lattices. Bragg Scattering.
domingo, 14 de febrero de 2010
sábado, 13 de febrero de 2010
Reciprocal Lattices (CONTINUACION)
Aim: To become familiar with the concept of the reciprocal lattice. It is very important and frequently used in the rest of the course to develop models and describe the physical properties of solids.
First we treat an alternative description of a lattice is in terms of a set of parallel identical planes. They are defined so that every lattice point belongs to one of these planes. The lattice can actually be described with an infinite number of such sets of planes. This description may seem artificial but we will see later that it is a natural way to interpret diffraction experiments in order to characterize the crystal structure. The figure below shows a 2-D lattice with three different sets of 1-D "planes" drawn.
The orientation of a plane can be specified by three integers, the so called Miller indices. The intercepts of one plane with the coordinate axes (the translation vectors of the lattice) are determined and normalized by division by the corresponding lattice constants. Then the reciprocals are taken and reduced to the set of smallest integers. In the figure above, the Miller indices for the three sets of "planes" are given as an example. Note also that the planes in the sets with lower Miller indices are spaced further apart (shaded areas depict the interplanar spacing). The Miller indices of a single plane or a set of planes are denoted (ijk). All planes with the same symmetry are denoted {ijk}. A direction in a crystal is denoted [uvw] and all equivalent directions (same symmetry) are . In cubic crystals [ijk] is orthogonal to (ijk).
Real lattices and reciprocal lattices
Lattices are periodic structures and position-dependent physical properties that depend on the structural arrangement of the atoms are also periodic. For example the electron density in a solid is a function of position vector r, and its periodicity can be expressed as ρ(r+T)=ρ(r), where T is a translation vector of the lattice. The periodicity means that the lattice and physical properties associated with it can be Fourier transformed. Since space is three dimensional, the Fourier analysis transforms it to a three-dimensional reciprocal space. Physical properties are commonly described not as a function of r, but instead as a function of wave vector ("spatial frequency" or k-vector) k. This is analogous to the familiar Fourier transformation of a time-dependent function into a dependence on (temporal) frequency. The lattice structure of real space implies that there is a lattice structure, the reciprocal lattice, also in the reciprocal space.
Reciprocal lattice vectors
The reciprocal lattice points are described by the reciprocal lattice vectors, starting from the origin G = m´ b1 + n´ b2 + o´ b3, where the coefficients are integers and the bi are the primitive translation vectors of the reciprocal lattice. From the definition of the bi, it can be shown that exp (iGT) = 1. This condition is actually taken as the starting point by Hoffman; it is required by the condition that the Fourier series must have the periodicity of the lattice. Hence, theFourier series of a function with the periodicity of the lattice can only contain the lattice vectors (spatial frequencies) G.
Since the Miller indices of sets of parallel lattice planes in real space are integers, we can interpret the coefficients (m´n´o´) as Miller indices (ijk). This leads to a physical relation between sets of planes in real space and reciprocal lattice vectors. Hence we write G = i b1 + j b2 + k b3. The reciprocal lattice vectors are labelled with Miller indices Gijk. Each point in reciprocal space comes from a set of crystal planes in real space (with some exceptions, for example (n00), (nn0) and (nnn) with n>1 in cubic systems; nevertheless they occur in the Fourier series and are necessary for a correct physical description). Consider the (ijk) plane closest to the origin. It cuts the coordinate axes in a1/i, a2/j and a3/k. A triangular section of the plane has these points in the corners. The sides of the triangle are given by the vectors (a1/i) - (a2/j) and analogously for the other two sides. Now the scalar product of Gijk with any of these sides is easily seen to be zero. Hence the vector Gijk is directed normal to the (ijk) planes. The distance between two lattice planes is given by the projection of for example a1/i onto the unit vector normal to the planes, i.e. dijk = (a1/i)•nijk, where dijk is the interplanar distance between two (ijk)-planes. The unit normal is just Gíjk divided by its length. Hence the magnitude of the reciprocal lattice vector is given by 2π/ dijk.
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visitar mi BLOG:
BIBLIOGRAFIA:
Get news, entertainment and everything you care about at Live.com. Check it out!
Etiquetas:
ORLANING COLMENARES
... Un poco de HISTORIA (Invariancia traslacional)
Ernesto Medina Dagger

Durante los siglos XIX y XX se le dio un gran impulso a la Física cuando se empezó a pensar en términos de simetrías. Una simetría se expresa matemáticamente como una invariancia (ausencia de cambios) bajo una operación como la de traslación espacial, temporal o, por ejemplo, una rotación. Si tomamos la figura de un cuadrado y la rotamos alrededor de su centro en 90 grados no podemos distinguir la orientación final de la original, el cuadrado es entonces invariante bajo una rotación de 90 grados. En la Física, las operaciones mencionadas dan origen respectivamente a la ley de conservación de energía (invariancia temporal), la ley de conservación de momentum (invariancia traslacional) y la de conservación de momento angular (invariancia rotacional). La presencia de todas estas invariancias juntas resulta en un mundo que no cambia en el tiempo, que es igual en todos los puntos del espacio y en todas las direcciones. Sin embargo, el mundo se pone interesante cuando ocurre el rompimiento de algunas de estas simetrías, lo cual da lugar a la formación de patrones o formas que varían de múltiples maneras en el espacio y el tiempo, lo que reconocemos intuitivamente como "orden" en la naturaleza. Los rompimientos de simetría dan lugar a muchos fenómenos con que convivimos, como la formación de cristales, los populares imanes o magnetos y la misma estructura que observamos del universo hoy en día. Sin el rompimiento de simetría no existirían los electrones, protones y neutrones que componen los átomos y por lo tanto los átomos mismos. No existiría la vida.
Un fenómeno supremamente importante, asociado al rompimiento de la simetría, es el surgimiento, paradójico, de una simetría exótica, la asociada a la invariancia de escalas. Formas y objetos que vemos a una escala de magnificación particular, se repiten a cualquier otra magnificación por encima o por debajo de la primera dando origen a patrones que son construidos en base a sí mismos. Esto es lo que conocemos como fractales y son las estructuras más ricas y bellas al ojo humano que ofrece la naturaleza.
Un fenómeno supremamente importante, asociado al rompimiento de la simetría, es el surgimiento, paradójico, de una simetría exótica, la asociada a la invariancia de escalas. Formas y objetos que vemos a una escala de magnificación particular, se repiten a cualquier otra magnificación por encima o por debajo de la primera dando origen a patrones que son construidos en base a sí mismos. Esto es lo que conocemos como fractales y son las estructuras más ricas y bellas al ojo humano que ofrece la naturaleza.
El estudio de simetrías y su rompimiento está hoy en el corazón de todos los campos de la física: la teoría de campos, la cosmología, la física de partículas, la física del estado sólido y fenómenos críticos.
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visitar mi BLOG:
Get news, entertainment and everything you care about at Live.com. Check it out!
Etiquetas:
ORLANING COLMENARES
Bravais Lattices (CONTINUACION)
Bravais lattices are the underlying structure of a crystal. A 3D Bravais lattice is defined by the set of vectors R
where the vectors a1 are the basis vectors of the Bravais lattice. (Do not confuse with a lattice with a basis.) Every point of a Bravais lattice is equivalent to every other point. In an elemental crystal, it is possible that the elemental ions are located at the vertices of a Bravais lattice. In general, a crystal structure will be a Bravais lattice with a basis. The symmetry group of a Bravais lattice is the group of translations through thelattice vectors together with some discrete rotation group about (any) one of thelattice points. In the problem set (Ashcroft and Mermin, problem 7.6) you will showthat this rotation group can only have 2-fold, 3-fold, 4-fold, and 6-fold rotation axes. There are 5 different types of Bravais lattice in 2D: square, rectangular, hexago-nal, oblique, and body-centered rectangular. There are 14 di erent types of Bravaislattices in 3D. The 3D Bravais lattices are discussed in are described in Ashcroft andMermin, chapter 7 (pp. 115-119). We will content ourselves with listing the Bravaislattices and discussing some important examples.
Bravais lattices can be grouped according to their symmetries. All but one canbe obtained by deforming the cubic lattices to lower the symmetry.
*Cubic symmetry: cubic, FCC, BCC.
*Tetragonal: stretched in one direction, a x a x c; tetragonal, centered tetragonal.
*Orthorhombic: sides of 3 di erent lengths a x b x c, at right angles to eachother; orthorhombic, base-centered, face-centered, body-centered.
*Monoclinic: One face is a parallelogram, the other two are rectangular; mono-clinic, centered monoclinic.
*Triclinic: All faces are parallelograms.
*Trigonal: Each face is an a x a rhombus.
*Hexagonal: 2D hexagonal lattices of side a, stacked directly above one another,with spacing c.
Examples:
*Simple cubic lattice: ai = a xi.
*Body-centered cubic (BCC) lattice: points of a cubic lattice, together with thecenters of the cubes = interpenetrating cubic lattices o set by 1/2 the body-diagonal.
Examples: Ba, Li, Na, Fe, K, Tl
*Face-centered cubic (FCC) lattice: points of a cubic lattice, together with the centers of the sides of the cubes, = interpenetrating cubic lattices o set by 1/2 a face-diagonal.
Examples: Al, Au, Cu, Pb, Pt, Ca, Ce, Ar.
*Hexagonal Lattice: Parallel planes of triangular lattices
Bravais lattices can be broken up into unit cells such that all of space can be recovered by translating a unit cell through all possible lattice vectors. A primitive unit cell is a unit cell of minimal volume. There are many possible choices of primitive unit cells. Given a basis, a1;a2;a3, a simple choice of unit cell is the region:
The volume of this primitive unit cell and, thus, any primitive unit cell is:
An alternate, symmetrical choice is the Wigner-Seitz cell: the set of all pointswhich are closer to the origin than to any other point of the lattice. Examples: Wigner-Seitz for square=square, hexagonal=hexagon (not parallelogram), oblique=distortedhexagon, BCC=octohedron with each vertex cut o to give an extra square face (A+Mp.74).
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visitar mi BLOG:
BIBLIOGRAFIA:
Discover the new Windows Vista Learn more!
Etiquetas:
ORLANING COLMENARES
Crystal momentum
In solid-state physics crystal momentum or quasimomentum is a momentum-like vector associated with electrons in a crystal lattice. It is defined by the associated wave vectors k of this lattice, according to
Pcristal = h.K
(where h is the reduced Planck's constant). Like regular momentum, crystal momentum frequently exhibits the property of being conserved, and is thus extraordinarily useful to physicists and materials scientists as an analytical tool.
Lattice symmetry origins
A common method of modeling crystal structure and behavior is to view electrons as quantum mechanical particles traveling through a fixed infinite periodic potential V(x) such that
V(x+a) = V(x)
where a is an arbitrary lattice vector. Such a model is sensible because (a) crystal ions that actually form the lattice structure are typically on the order of tens of thousands of times more massive than than electrons, making it safe to replace them with a fixed potential structure, and (b) the macroscopic dimensions of a crystal are typically far greater than a single lattice spacing, making edge effects negligible. A consequence of this potential energy function is that it is possible to shift the initial position of an electron by any lattice vector a without changing any aspect of the problem, thereby defining a discrete symmetry. (Speaking more technically, an infinite periodic potential implies that the lattice translation operator T(a) commutes with the Hamiltonian, assuming a simple kinetic-plus-potential form.)
These conditions imply Bloch's theorem, which states in terms of equations that
These conditions imply Bloch's theorem, which states in terms of equations that
or in terms of words that an electron in a lattice, which can be modeled as a single particle wave function ψ(x), finds its stationary state solutions in the form of a plane wave multiplied by a periodic function u(x). The theorem arises as a direct consequence of the aforementioned fact that the lattice symmetry translation operator commutes with the system's Hamiltonian.
One of the notable aspects of Bloch's theorem is that it shows directly that steady state solutions may be identified with a wave vector k, meaning that this quantum number remains a constant of motion. Crystal momentum is then conventionally defined by multiplying this wave vector by Planck's constant:
One of the notable aspects of Bloch's theorem is that it shows directly that steady state solutions may be identified with a wave vector k, meaning that this quantum number remains a constant of motion. Crystal momentum is then conventionally defined by multiplying this wave vector by Planck's constant:
Pcristal = h.K
While this is in fact identical to the definition one might give for regular momentum (for example, by treating the effects of the translation operator by the effects of a particle in free space), there are important theoretical differences. For example, while regular momentum is completely conserved, crystal momentum is only conserved to within a lattice vector, i.e., an electron can be described not only by the wave vector k, but also with any other wave vector k' such that
k* = k + K
where K is an arbitrary reciprocal lattice vector. This is a consequence of the fact that the lattice symmetry is discrete as opposed to continuous, and thus its associated conservation law cannot be derived using Noether's theorem.
It is tempting to treat a phonon with wave vector as though it has a momentum, by analogy to photons and matter waves. This is not entirely correct, for is not actually a physical momentum; it is called the crystal momentum or pseudomomentum. This is because is only determined up to multiples of constant vectors, known as reciprocal lattice vectors. For example, in our one-dimensional model, the normal coordinates and are defined so that
It is usually convenient to consider phonon wave vectors which have the smallest magnitude in their "family". The set of all such wave vectors defines the first Brillouin zone. Additional Brillouin zones may be defined as copies of the first zone, shifted by some reciprocal lattice vector.
It is interesting that similar consideration is needed in analog-to-digital conversion where aliasing may occur under certain conditions.
It is interesting that similar consideration is needed in analog-to-digital conversion where aliasing may occur under certain conditions.
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visitar mi BLOG:
BIBLIOGRAFIA:
Invite your mail contacts to join your friends list with Windows Live Spaces. It's easy! Try it!
Etiquetas:
ORLANING COLMENARES
Brillouin zone
In mathematics and solid state physics, the first Brillouin zone is a uniquely defined primitive cell in reciprocal space. The boundaries of this cell are given by planes related to points on the reciprocal lattice. It is found by the same method as for the Wigner–Seitz cell in the Bravais lattice. The importance of the Brillouin zone stems from the Bloch wave description of waves in a periodic medium, in which it is found that the solutions can be completely characterized by their behavior in a single Brillouin zone.
Taking surfaces at the same distance from one element of the lattice and its neighbours, the volume included is the first Brillouin zone. Another definition is as the set of points in k-space that can be reached from the origin without crossing any Bragg plane. Equivalently, this is the Voronoi cell around the origin of the reciprocal lattice.
There are also second, third, etc., Brillouin zones, corresponding to a sequence of disjoint regions (all with the same volume) at increasing distances from the origin, but these are used more rarely. As a result, the first Brillouin zone is often called simply the Brillouin zone. (In general, the n-th Brillouin zone consists of the set of points that can be reached from the origin by crossing exactly n − 1 distinct Bragg planes.)
A related concept is that of the irreducible Brillouin zone, which is the first Brillouin zone reduced by all of the symmetries in the point group of the lattice.
The concept of a Brillouin zone was developed by Léon Brillouin (1889-1969), a French physicist.
Taking surfaces at the same distance from one element of the lattice and its neighbours, the volume included is the first Brillouin zone. Another definition is as the set of points in k-space that can be reached from the origin without crossing any Bragg plane. Equivalently, this is the Voronoi cell around the origin of the reciprocal lattice.
There are also second, third, etc., Brillouin zones, corresponding to a sequence of disjoint regions (all with the same volume) at increasing distances from the origin, but these are used more rarely. As a result, the first Brillouin zone is often called simply the Brillouin zone. (In general, the n-th Brillouin zone consists of the set of points that can be reached from the origin by crossing exactly n − 1 distinct Bragg planes.)
A related concept is that of the irreducible Brillouin zone, which is the first Brillouin zone reduced by all of the symmetries in the point group of the lattice.
The concept of a Brillouin zone was developed by Léon Brillouin (1889-1969), a French physicist.
Para ahondar un poco mas en este tema se tiene:



Para resumir un poco:
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visitar mi BLOG:
BIBLIOGRAFIA:
Get news, entertainment and everything you care about at Live.com. Check it out!
Etiquetas:
ORLANING COLMENARES
viernes, 12 de febrero de 2010
Bragg Scattering
SCATTERING DIFUSO DE NEUTRONES EN MATERIALES INORGÁNICOS LUMINISCENTES
En los últimos años, nuestro trabajo de investigación se ha concentrado en avanzar en el conocimiento de los aspectos estructurales y espectroscópicos de los compuestos del tipo elpasolitas, estequiométricas y no estequiométricas, donde Ln(+3) y Ln(+3) son iones lantanidos trivalentes positivos y Z(-1) representa un ion halogenuro.
Para este objetivo, se han identificados nuevas rutas de síntesis y hemos implementado técnicas de análisis instrumental y de caracterización espectroscópica, tanto al nivel de investigación básica como aplicada.
Las técnicas de difracción de rayos X (DRX), difracción de neutrones y el scattering difuso de neutrones, proporcionan datos estructurales esenciales para un conocimiento acabado de la estructura cristalina de este tipo de estructuras cristalinas. En este trabajo, realizado con la infraestructura del reactor nuclear, del centro de estudios nucleares de la Reina (CCHEN), se presenta la técnica de scattering difuso de neutrones, el procedimiento de calibración y algunos estudios exploratorios con una aplicación concreta a la elpasolita estequiométrica de Holmio (+3)
En física del estado sólido, el estudio de procesos radiativos y no radiativos corresponde a un área emergente de interés renovado, esencialmente por el advenimientos de técnica de detección instrumental. Existen avances importantes tanto desde el punto de vista estructural y espectroscópico como también en el desarrollo de nuevos formalismos de cálculo. Resultan ser de gran interés, abordar el análisis de cristales puros y dopados de simetría cúbica, para los cuales existe una masa importante de datos experimentales, obtenidos utilizando técnicas de espectroscopía lineal (Infrarrojo y Luminiscencia) y no lineal (Raman y espectroscopía de dos fotones). Diversos son nuestros objetivos en esta área del conocimiento: diseño y análisis de nuevas rutas de síntesis, caracterización estructural (difracción de rayos X y difracción de neutrones) , desarrollo de formalismos de cálculo generalizados en la aproximación molecular, (desprecio de las interacciones entre las vibraciones internas y externas) y en dinámica de cristales (sistemas puros y dopados).
Los sistemas dopados son complejos, y esta dificultad teórico y experimental aumenta en forma importante al sustituir los ligandos monodentados por ligandos polidentados. La estrategia a seguir consiste en la obtención de datos experimentales nuevos y reproducibles, lo cual nos permita progresar en la formulación de modelos teóricos de envergadura aplicables a física del estado sólido y en particular a dinámica de cristales. Se trata, de considerar en forma explícita la inclusión de interacciones de corto alcance (campos de fuerzas vibracionales) y de largo alcance (sumas cristalinas sobre el espacio directo y recíproco) en la representación de la matriz dinámica con la posterior resolución de la ecuación dinámica.
Nuestro interés inmediato, se ha focalizado en el estudio de las elpasolitas estequiométricas, , donde representa un ion lantánido trivalente positivo y el cristal es clasificado de acuerdo a la simetría del grupo espacial . Es de conocimiento general, que el scattering difuso de neutrones se favorece por la existencia de defectos y vacancias en sólidos policristalinos, como ocurre, por ejemplo, en los sistemas en forma de polvos, cerámicos, aleaciones metálicas y compuestos de última generación y de gran interés tecnológico. Es de nuestro interés aplicar este tipo de técnicas experimentales al estudio de las elpasolitas estequiométricas y no estequiométricas del tipo y , respectivamente.
One way of experimentally probing a condensed matter system involves scattering a photon or neutron the system and studying the energy and angular dependence of the resulting cross-section. Crystal structure experiments have usually been done with X-rays.
ELEMENTOS INTRODUCTORIOS
Al hacer incidir un haz de neutrones en un blanco, ocurren tres fenómenos competitivos; scattering, transmisión y absorción de neutrones. Como resultado del alto poder de penetración de los neutrones, éstos interactúan con la totalidad de la muestra.
Resulta ser factible separar experimentalmente, los fenómenos de transmisión y absorción de los procesos de scattering. Al incidir los neutrones en la muestra, la sección eficaz de choque se define, como la razón entre el número de interacciones posibles por segundo y el flujo de los neutrones incidentes. La magnitud contiene toda la información relacionada con la interacción entre los neutrones y los núcleos del blanco, como una función de los estados iniciales y finales del sistema neutrón-blanco.
La amplitud de Scattering para un conjunto de núcleos está dada por la suma de las amplitudes individuales de dispersión de todos y cada uno de los estados permitidos multiplicados por un factor de fase , aproximación de Born.
En el caso elástico tenemos , y los estados son de la forma:

donde, Rn es el vector de posición del átomo n-ésimo con respecto del origen. En virtud de lo anterior, la diferencial de la sección eficaz es:
El análisis de la expresión anterior para , contiene dos contribuciones: incoherente (scattering difuso) y coherente (scattering de Bragg), respectivamente.
SCATTERING DIFUSO DE NEUTRONES
a) Montaje experimental
El instrumento de medición, utiliza el haz radial sur de neutrones del reactor del Centro de Estudios Nucleares La Reina (el cual posee una potencia de operación de 5 MW) y nos permite medir la intensidad de scattering difuso (incoherente) de los neutrones que interactuan con la muestra. Esta instrumentación ésta basada en la técnica de tiempo de vuelo de los neutrones y proporciona una distribución de intensidades de scattering en función de la longitud de ondas incidente y el ángulo de detección de los neutrones scattereados. Se utiliza un filtro de Bismuto (monocristalino orientado), el cual elimina los neutrones rápidos y radiación gamma. Adicionalmente se utiliza un filtro de Berilio (policristalino) cuya función es remover del haz, los neutrones con longitud de onda menor a 3.95Å, eliminando de esta forma, la contribución de Bragg. Los dos filtros están a la temperatura de a 77 K, lo anterior nos permite obtener un haz de neutrones caracterizado por tener rango de longitudes de ondas 4Å -7Å. A la salida de los filtros, se utiliza un disco absorbente de neutrones (Al-Cd) como pulsador, el cual posee seis agujeros equidistantes transparentes a los neutrones. Este disco gira a 6000 rpm proporcionando pulsos cada 1,75 milisegundos, permitiendo el paso de trenes de energía, los cuales interactuan con la muestra. El flujo en la posición de la muestra alcanza un valor promedio del orden de . Finalmente, los neutrones dispersados por la muestra son registrados en un banco de doce detectores de BF3 y la información así obtenida, es procesada por la electrónica asociada (periférico) al sistema de medición.
b) Calibración y Aplicación
El procedimiento se realiza de acuerdo a la siguiente forma: (a) Se utiliza Vanadio de alta pureza (99,99%) como muestra de calibración. (b) Se procede a calibrar la electrónica de cada uno y todos los doce detectores, permitiendo la normalización de la señal registrada por los detectores. Para efectos ilustrativos, se muestra la normalización de tres detectores, se presenta un resumen del proceso de calibración de seis detectores.
Se ha sintetizado el cristal estequiométrico , utilizando una reacción química seguida de una reacción de estado sólido, obteniéndose un parámetro de red a=10,7234Å , esto se realizó por medio de las técnicas de DRX y difracción de neutrones, seguido del refinamiento de estructuras por el método de Rietvelt.
Estos datos estructurales demuestran que se trata de una estructura cúbica perteneciente al grupo espacial .
c) Estudio Exploratorio
En este trabajo reportamos datos pioneros en cuanto a la distribución de energías, por el tiempo de vuelo para la elpasolita [Ver Figura 4, correspondiente al espectro de este cristal puro]. Este espectro de distribución de energías (exploratorio), nos permitirá estudiar el scattering originado por los defectos cristalinos, que aparecen para una amplia variedad de elpasolitas dopadas de este mismo ion lantánido. Este fenómeno cobra importancia en la medida que los iones lantánidos utilizados en el dopaje químico, por definición aleatoria, presenten distribuciones electrónicas y nucleares semejantes, preservando la simetría traslacional del cristal anfitrión. No obstante, los espectros de distribución de energías presentarán simultáneamente intensidades distintas y corrimientos, lo anterior como resultado del procedimiento de dopaje. A bajas temperaturas, una proyección natural de este trabajo nos conduce a observaciones de transiciones nucleares, para lo cual será necesario optimizar el sistema de medición experimental.
CONCLUSIONES
El trabajo experimental exploratorio demuestra que el objetivo central de calibración y puesta en marcha del instrumento ha sido logrado, obteniéndose resultados pioneros en el caso de sistemas de alto interés tanto básico como tecnológico. Esta técnica es complementaria a todas las otras facilidades experimentales que utilizan haces de neutrones (difracción de neutrones, scattering inelástico de neutrones y scattering de neutrones con ángulos pequeños). Las implicancias futuras en aspectos estructurales (descensos de simetría) nos permitirá acceder a una nueva masa de datos experimentales, y nos posibilitará el estudio de la interacción electrón-fonón y otros relacionados. De particular importancia será analizar las curvas de dispersión de fonones en cristales cúbicos y ligeramente distorsionados, para distintas direcciones de polarización.
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visitar mi BLOG:
BIBLIOGRAFIA:
Connect to the next generation of MSN Messenger Get it now!
Etiquetas:
ORLANING COLMENARES
More Realistic Phonon Spectra
Van Hove singularities and nonadiabatic effects in superconductivity
Abstract
The breakdown of Migdal's theorem implies the generalization of the electronphonon theory of superconductivity to include various nonadiabatic effects. The reduced electronic screening due to the small carrier density and the presence of a van Hove singularity forthe optimized cuprates complete a new scenario for both the superconductive and the normalstate. In particular the van Hove singularity can be related to highest values of Tc, to thereduction of the isotope effect and to the linear resistivity.
A common feature of all unconventional superconductors: cuprates, fullerene compoundsand organics is the extremely small carrier density, one or two orders of magnitudes smaller than in usual metals. This situation has two fundamental consequences: on the one handelectrons (or holes) are strongly correlated and, on the other hand, it locates these systems outside the range of validity of Migdal's theorem. The small carrier density corresponds to a very unfavorable situation with respect to the usual BCS-Eliashberg theory becausethe average density of states (DOS) is rather small and the residual Coulomb repulsion is certainly larger than in usual superconductors. An additional element which is characteristic of the oxides is the presence of a van Hove singularity (vHs) in the DOS near the Fermi level. In our opinion, however, there is a hierarchy of importance between the three elements previously mentioned. Namely the breakdown of Migdal's theorem requires the generalization of the Eliashberg equations to the nonadiabatic regime. This is what we have done recently, and the main result is that, in a favorable situation of positive vertex corrections etc., the nonadiabatic effects can appreciably raise Tc and lead to a number of new effects for both the superconductive and normal state. This result is particularly appealing because it allows us to understand the positive role of the small carrier density in these systems. Within this frame work of nonadiabatic superconductivity the role of electronic correlations and the vHs is to locate the system in a favorable range of parameters. In this perspective the main effect of electronic correlations is to favor small momentum scattering, leading to positive nonadiabatic effects. As for the vHs the situation is more delicate because, apartfrom the value of the total bandwidth, the vHs intrinsically implies a breakdown of Migdal'stheorem and it can only be studied consistently by including the nonadiabatic effects.In this letter we focus in particular on the interplay between nonadiabatic effects and vHs.The effect of electronic correlations is parameterized by a modulation of the electron-phononcoupling as a function of momentum. We show that with these elements it is possibleto understand in a coherent way many properties of both the superconductive and normalstate. In particular the small carrier density can be related to an enhancement of Tc via thenonadiabatic effects. The vHs ampli¯es these effects and provides a further enhancement of Tcassociated to a decrease of the isotope effect. In addition, for the normal state, the above elements lead to a rather complex generalization of the usual Fermi liquid whose properties can reproduce many of the observed anomalies. The Migdal parameter is m = D=EF, where D is the Debye frequency and EF the Fermienergy. The usual Eliashberg theory of SC includes the self-energy effects to all orders in (dimensionless el-ph coupling) in the limit m = 0. The extension of Eliashberg equations tothe nonadiabatic regime includes the ¯rst-order effects with respect to the parameter and changes qualitatively the structure of the theory. In some sense this change is of thesame order as the change from BCS to Eliashberg, once one includes the self-energy effects. Inaddition this new theory preserves, in some sense, the Fermiliquid nature of the system butin a more complex phenomenology. Which seems actually to be the situation in high-Tc materials.An essential element in the nonadiabatic theory of superconductivity is the structure of the vertex correction function
expressed in usual Matsubara notations. The vertex function PV depends essentially on theexchanged frequency and the momentum q, and contains as parameters the phonon frequency 0 (single Einstein mode) and the bandwidth E = 2EF, considering thehalf-¯lled case. A detailed study of this function shows that it is positive in the dynamical limit (smallq and large), while it is negative in the static limit (large q and small). This leads tothe result that small q scattering is associated to positive vertex corrections and there for eto an enhancement of Tc. This result shows that the common opinion that vertex corrections are, in general, negative, is actually incorrect. The predominance of smallq scattering is a characteristic of strongly correlated electrons. In addition, the presenceof a vHs strongly enhances the phase space for small q scattering within the same vHs andtherefore leads to a further enhancement of Tc. In order to study the effect of a vHs on the vertex corrections, we consider the simpledispersion on the saddle point to which there corresponds a logarithmic, where E is the bandwidth and N0 is the average DOS over the wholeband. We consider the Fermi level just on the singularity and assume an isotropic dispersion,so that the vertex function will depend only on q = jqj. We also consider only the case n = 0in order to derive analytical expressions that have also been tested numerically.
In a normal situation vertex corrections are of order l x m. In the presence of a vHs we obtain instead
The nonadiabatic generalization of Eliashberg equations implies the inclusion of vertexcorrections and of the cross phonon diagram. We include the complex dependence ofvertex (PV) and cross (PC) diagrams on momentum and frequency by performing a weighted average on q and. In order to reproduce the effect of electronic correlations, we introduce anupper momentum cut qc in the structure of the el-ph interaction g(q). In addition thisfunction is normalized in such a way that the total coupling strength is constant for different qc. The generalized equations for the superconducting instability can be written as
The gap equation can be solved analytically by factorizing the gap kernel Dn-m = Dn x Dm and the final expression for the critical temperature in the nonadiabatic van Hove scenariois
The behavior of Tc as a function of (l) is reported for a fixed value of m=0,1. It shows a remarkable increase of the critical temperature due to the vertex and cross terms with respect to the Migdal-Eliashberg adiabatic case, especially for small values of (l). Note that such an enhancement is evident also for relatively small values of m (m=0,1). Moreover, the ratio of the nonadiabatic vs. the adiabatic Tc would be increased even more by the introduction
Behavior of the resistivity as a function of temperature derived from Boltzmann theory and including both the normal and Umklapp scattering.
In our perspective, therefore, all the HTSC, i.e. oxides, fullerene and organics, would
be very unfavorable systems from the BCS-Eliashberg point of view, corresponding to no
superconductivity at all, or with extremely small values of Tc =1K. The nonadiabatic effects
lead to a new structure of the theory in which the value of Tc can be strongly enhanced. This
is the main effect that brings the values of the critical temperature in the range Tc =10-40K.
In the optimized cuprates the vHs gives a further element to this scheme and can increase Tc
above 100K.
This new scenario of nonadiabatic behavior and vHs for the optimized cuprates has also
important implications for the properties of the normal state that corresponds to a complex
type of Fermi liquid. For example, one of the most puzzling properties of the optimized
cuprates is the linear resistivity over a broad range of temperatures. This trend was shown
to be recovered in the van Hove scenario with purely electronic interactions. Anyway,
it should be pointed out that this result rests just on the distribution of density of states
on the Fermi surface, presenting a 1/k divergence of the DOS along the asymptotes of the
Fermi surface crossing point. This gives rise to extra phase space effects that change the
temperature dependence. Actually, we would like to point out that these
arguments hold in full generality also for the electron-phonon scattering.
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visita mi BLOG:
BIBLIOGRAFIA:
Invite your mail contacts to join your friends list with Windows Live Spaces. It's easy! Try it!
Etiquetas:
ORLANING COLMENARES
Local Magnetoplasmon Modes of a Semiconductor
Para este caso, por ser información precisa, seleccione el tema de esta manera.
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visitar mi BLOG:
BIBLIOGRAFIA:
Invite your mail contacts to join your friends list with Windows Live Spaces. It's easy! Try it!
Etiquetas:
ORLANING COLMENARES
Debye theory
Debye Specific Heat
By associating a phonon energy
By associating a phonon energy
with the vibrational modes of a solid, where vs is the speed of sound in the solid, Debye approached the subject of the specific heat of solids. Treating them with Einstein-Bose statistics, the total energy in the lattice vibrations is of the form
This can be expressed in terms of the phonon modes by expressing the integral in terms of the mode number n.
Here the factor 3p/2 comes from three considerations. First, there are 3 modes associated with each mode number n: one longitudinal mode and two transverse modes. Then you get a factor of 4p2 from integrating over the angular coordinates, treating the mode number n as the radius vector. Finally you constrain the integral to the quadrant in which all the components of n are positive, giving a factor of 1/8: the product of those is 3p/2.
The usual form of the integral is obtained by making the substitution
and the limit on the integral in terms of x is obtained from
where the constant TD is here introduced. It is called the Debye temperature and is a constant associated with the highest allowed mode of vibration.
When all the constants are put in, the integral takes the form:
The Debye specific heat expression is the derivative of this expression with respect to T. The integral cannot be evaluated in closed form, but numerical evaluation of the integral shows reasonably good agreement with the observed specific heats of solids for the full range of temperatures, approaching the Dulong-Petit Law at high temperatures and the characteristic T3 behavior at very low temperatures.
The specific heat expression which arises from Debye theory can be obtained by taking the derivative of the energy expression above.
This expression may be evaluated numerically for a given temperature by computer routines.
Since the Debye specific heat expression can be evaluated as a function of temperature and gives a theoretical curve which has a specific form as a funtion of T/TD, the specific heats of different substances should overlap if plotted as a function of this ratio. At left below, the specific heats of four substances are plotted as a function of temperature and they look very different. But if they are scaled to T/TD, they look very similar and are very close to the Debye theory.
THEORY
Since a solid can be modelled as a collection of independent oscillators, we can obtain the energy in thermal equilibrium using the Planck distribution function:
where s = 1; 2; 3 are the three polarizations of the phonons and the integral is overthe Brillouin zone.This can be rewritten in terms of the phonon density of states, g(w) as:
The total number of states is given by:
The total number of normal modes is equal to the total number of ion degrees offreedom. For a continuum elastic medium, there are two transverse modes with velocity vtand one longitudinal mode with velocity vl. In the limit that the lattice spacing is very small, a ---> 0, we expect this theory to be valid. In this limit, the Brillouin zoneis all of momentum space, so
In a crystalline solid, this will be a reasonable approximation to g(w) where the only phonons present will be at low energies, far from the Brillouinzone boundary. At high temperatures, there will be thermally excited phonons near the Brillouin zone boundary, where the spectrum is de nitely not linear, so we cannot use the continuum approximation. In particular, this g(w) does not have a nite integral, which violates the condition that the integral should be the total number of degrees of freedom.A simple approximation, due to Debye, is to replace the Brillouin zone by a sphere of radius kD and assume that the spectrum is linear up to kD. In other words, Debyeassumed that:
Here, we have assumed, for simplicity, that vl = vt and we have written D is chosen so that
The T3 contribution to the speci c heat of a solid is often the most important contribution to the measured specific heat.
The high-temperature specific heat is just kB=2 times the number of degrees of free-dom, as in classical statistical mechanics.At high-temperature, we were guaranteed the right result since the density ofstates was normalized to give the correct total number of degrees of freedom. Atlow-temperature, we obtain a qualitatively correct result since the spectrum is linear. To obtain the exact result, we need to allow for longitudinal and transverse velocities which depend on the direction, since rotational invariance is not present.Debye's formula interpolates between these well-understood limits. For lead, which is soft, while fordiamond, which is hard.
ORLANING COLMENARES
ELECTRONICA DEL ESTADO SOLIDO
Visitar mi BLOG:
BIBLIOGRAFIA:
Get news, entertainment and everything you care about at Live.com. Check it out!
Etiquetas:
ORLANING COLMENARES
Suscribirse a:
Comentarios (Atom)