Phonon:
In physics, a phonon is a quantum of energy, relating to a mode of vibration occurring in a rigid crystal lattice, such as the atomic lattice of a solid. It was introduced by Russian scientist Igor Tamm. The study of phonons is an important part of solid state physics, because phonons play a major role in many of the physical properties of solids, including a material's thermal and electrical conductivities. In particular, the properties of long-wavelength phonons give rise to sound in solids—hence the name phonon from the Greek φωνή (phonē) = voice. In insulating solids, phonons are also the primary mechanism by which heat conduction takes place.
Phonons are a quantum mechanical version of a special type of vibrational motion, known as normal modes in classical mechanics, in which each part of a lattice oscillates with the same frequency. These normal modes are important because, according to a well-known result in classical mechanics, any arbitrary vibrational motion of a lattice can be considered as a superposition of normal modes with various frequencies (compare Fourier transform); in this sense, the normal modes are the elementary vibrations of the lattice. Although normal modes are wave-like phenomena in classical mechanics, they acquire certain particle-like properties when the lattice is analysed using quantum mechanics (see wave-particle duality.)
Phonons are a quantum mechanical version of a special type of vibrational motion, known as normal modes in classical mechanics, in which each part of a lattice oscillates with the same frequency. These normal modes are important because, according to a well-known result in classical mechanics, any arbitrary vibrational motion of a lattice can be considered as a superposition of normal modes with various frequencies (compare Fourier transform); in this sense, the normal modes are the elementary vibrations of the lattice. Although normal modes are wave-like phenomena in classical mechanics, they acquire certain particle-like properties when the lattice is analysed using quantum mechanics (see wave-particle duality.)
Consider the lattice of ions in a solid. Suppose the equilibrium positions of the ions are the sites Ri. Let us describe small displacements from these sites by a displacement eld u(Ri). We will imagine that the crystal is just a system of masses connected by springs of equilibrium length a. Before considering the details of the possible lattice structures of 2D and 3D crystals, let us consider the properties of a crystal at length scales which are much larger than the lattice spacing; this regime should be insensitive to many details ofthe lattice. At length scales much longer than its lattice spacing, a crystalline solid can be modelled as an elastic medium. We replace u(Ri) by u(r) (i.e. we replace the lattice vectors, Ri, by a continuous variable, r). Such an approximation is valid atlength scales much larger than the lattice spacing, a, or, equivalently, at wave vectors q<<2pi/a.In 1D, we can take the continuum limit of our model of masses and springs:
where p is the mass density and B is the bulk modulus. The equation of motion,
has solutions, with w=
The generalization to a 3D continuum elastic medium is:
Above, we introduced the concept of the polarization of a phonon. In 3D, the displacements of the ions can be in any direction. The two directions perpendicular to k are called transverse. Displacements along k are called longitudinal.The Hamiltonian of this system,
can be rewritten in terms of creation and annihilation operators,
Inverting the above de nitions, we can express the displacement u(r) in terms ofthe creation and annihilation operators:
s = 1; 2; 3 corresponds to the longitudinal and two transverse polarizations. Acting with uk either annihilates a phonon of momentum k or creates a phonon of momentum k.
The allowed k values are determined by the boundary conditions in a nite system. For periodic boundary conditions in a cubic system of size V = L3, the allowed k's are 2pi/L (n1; n2; n3). Hence, the k-space volume per allowed k is (2pi)3=V . Hence, wecan take the infinite-volume limit by making the replacement:
Elastic Theory of Adsorbate Vibrational Relaxation
Abstract: An analytical theory is presented for the damping of low-frequency adsorbate vibrations via resonant coupling to the substrate phonons. The system is treated classically, with the substrate modeled as a semi-infinite elastic continuum and the adsorbate overlayer modeled as an array of point masses connected to the surface by harmonic springs. The theory provides a simple expression for the relaxation rate in terms of fundamental parameters of the system: $\gamma = m\bar{\omega}_0^2/A_c \rho c_T$, where m is the adsorbate mass. This expression is strongly coverage dependent, and predicts relaxation rates in excellent quantitative agreement with available experiments. For a half-monolayer of carbon monoxide on the copper (100) surface, the predicted damping rate of in-plane frustrated translations is $0.50\times 10^{12}$~s$^{-1}$, as compared to the experimental value of $(0.43\pm0.07)\times 10^{12}$ s$^{-1}$. Furthermore it is shown that, for all coverages presently accessible to experiment, adsorbate motions exhibit collective effects which cannot be treated as stemming from isolated oscillators.
ORLANING COLMENARES
C.I.V.- 18.991.089
ELECTRONICA DEL ESTADO SOLIDO
Visitar mi BLOG:
Bibliografia:
Get news, entertainment and everything you care about at Live.com. Check it out!





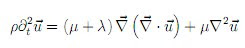




No hay comentarios:
Publicar un comentario